Linear Momentum Questions with Solutions

Linear momentum questions with solutions and explanations are presented. These questions may be used to practice for the SAT physics test.

Questions

If the speed and mass of an object are doubled, which of the following is true?
A) The momentum of the object is doubled
B) The kinetic energy of the object is doubled
C) The momentum of the object is quadrupled
D) The kinetic energy of the object is multiplied by 8
E) The momentum and the kinetic energy of the object are quadrupled

Two object A and B of velocities v1 and v2 have momenta with equal magnitude. If |v1| < |v2|, which of the following is true?
A) The two objects have equal kinetic energies
B) The two objects have equal masses
C) The kinetic energy of A is greater than the kinetic energy of B
D) The kinetic energy of B is greater than the kinetic energy of A
E) Mass of object A is greater than mass of object B

Two objects A and B have velocities v1 and v2 and masses m1 and m2. If |v1| < |v2| and the two objects have equal kinetic energies, which of the following is true?
A) The two objects have momenta with equal magnitudes
B) The magnitude of the momentum of A is greater than the magnitude of the momentum of B
C) The magnitude of the momentum of A is smaller than the magnitude of the momentum of B
D) The two objects have equal masses
E) Mass of object A is smaller than mass of object B

How long does it take a 2000-Kg car to stop from a velocity of 35 m/s if a braking force of 4000 Newtons is used?
A) 35 s
B) 70 s
C) 8.75 s
D) 10 s
E) 17.5 s

Object A of mass m1 is moving at a velocity v1 to the right. It collides and sticks to object B of mass m2 moving in the same direction as object A with a velocity v2. After collision, the two objects have a velocity equal to (1/2)(v1 + v2). What is the relationship between m1 and m2?
A) m1 > m2
B) m1 < m2
C) m1 = 2 m2
D) m1 = m2
E) m1 = (1/2) m2

A 2-Kilogram object slides, on a smooth surface, towards the north at a velocity of 5 meters per second. The object hits a fixed pole and is deflected from north to east by an angle of 60° and has a velocity of 5 meters per second. The change in the magnitude of the northward component of the moment of the object is
A) - 15 Kg.m/s
B) - 10 Kg.m/s
C) -5 Kg.m/s
D) 0 Kg.m/s
E) 5 Kg.m/s

An object of mass 4 Kg is moving towards the east at a velocity of 6 meters per second. It collides and sticks to a 6-Kg object moving with a velocity of 5 meters per second in the same direction. How much kinetic energy was lost in the collision?
A) 1.2 J
B) 4.25 J
C) 0 J
D) 2.4 J
E) 5 J

Two objects A and B of masses 1 and 3 Kg are held by a compressed massless spring and are at rest. When the spring is released, object A moves to the left with a velocity of 9 m/s. What is the velocity (magnitude and direction) of object B?
A) 3 m/s to the left
B) 3 m/s to the right
C) 9 m/s to the left
D) 9 m/s to the right
E) 4.5 m/s to the left

Which of the following statements is true?
A) In an elastic collision, only momentum is conserved.
B) In an inelastic collision, both momentum and kinetic energy are conserved.
C) In an elastic collision, only kinetic energy is conserved.
D) In any collision, momentum is conserved.
E) In any collision, both momentum and kinetic energy are conserved.

An object A of mass m1 is moving at a speed v1 in a straight line to the right. Another object B of mass m2 is moving to the left in the same path as object A but in the opposite direction. What would be the speed of object B be so that when the two objects collide they stick to each other and stop?
A) m1 |v1| / (m1 + m2)
B) m1 |v1| / m2
C) (m1 + m2) |v1| / m1
D) m2 |v1| / m1
E) m2 |v1| / (m1 + m2)

Solutions to Above Questions

p = m v
k = (1/2) m v²
M = 2 m and V = 2 v (mass and velocity doubled)
P = M V = (2m)(2v) = 4 m v momentum is quadrupled.
K = (1/2) M V² = (1/2) (2 m) (2 v) ² = (1/2) 8 m v² : kinetic energy is multiplied by 8
Answer: D

Equal momenta
|p1| = m1 |v1| and |p2| = m2 |v2| , magnitude of momenta of objects A and B
Let K1 = (1/2) m1 |v1|² and K2 = (1/2) m2 |v2|² , kinetic energies of objects A and B
We know that
|v1| < |v2|
Multiply both sides of the above inequality by (1/2) |p1| and (1/2) |p2| taking into account that |p1| = |p2|
(1/2) |p1| |v1| < (1/2) |p2| |v2|
Substitute |p1| and |p2| by their expressions given above to obtain
(1/2) m1 |v1| |V1| < (1/2) m2 |v2| |v2|
which gives
K1 < K2
The kinetic energy of B is greater than that of A
Answer: D

Equal kinetic energies
K1 = (1/2) m1 |v1|² , K2 = (1/2) m2 |v2|² , kinetic energies of objects A and B are equal
K1 = K2 given
Let |p1| = m1 |v1| and |p2| = m2 |v2| , the momenta of objects A and B
We know that
|v1| < |v2|
Divide the numerator and denominator in the above by K1 and K2 (note K1 = K2), to obtain
|v1| / K1 < |v2| / K2
Which gives
K1 / |v1| > K2 / |v2|
Substitute K1 and K2 by their expressions given above
(1/2) m1 |v1|² / |v1| > (1/2) m2 |v2|² / |v2|
Simplify to obtain
m1 |v1| > m2 |v2|
Which gives |p1| > |p2|
Answer: B

The brakes in a car are used to stop the car hence to change the momentum of the car from some value to zero. The relationship between an applied force to an object of mass m and the change of its momentum in physics is given by
F Δt = Δp = m (vf - vi) , vf final velocity and vi initial velocity, Δ t is the time during which force F is applied.
in magnitude
|F| Δ t = m |vf - vi| , assuming vf and vi either in same or opposite directions.
Δ t
Δ t = 2000 |0 - 35| / 4000 = 17.5 seconds
Answer: E

Momentum before collision: m1 v1 + m2 v2
Momentum after collision: (m1 + m1)(1/2)(v1 + v2)
Momentum are conserved m1 v1 + m2 v2 = (m1 + m1)(1/2)(v1 + v2)
Multiply all terms by 2, expand and simplify
m1 v1 - m1 v2 + m2 v2 - m2 v1 = 0
factor
m1 (v1 - v2) - M2(v1 - v2) = 0
(m1 - m2)(v1 - v2) = 0
taking into account that v1 is not equal to v2, solve the above equation to obtain
m1 = m2
Answer: D

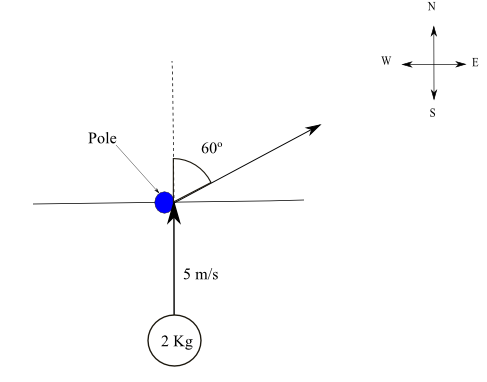
Momentum before hitting the pole: p1 = m v = 2 × 5 = 10 K.m/s one component (only) to the north
Momentum after hitting the pole: 2 components: to the north p2n = 2 × 5 cos (60°) to the east: p2e = 2 × 5 sin (60°)
Change in magnitude of components: p2n - p1 = 2 × 5 cos (60°) - p1 = 5 - 10 = - 5 Kg.m/s
Answer: C

Momentum before collision: p1 = 4×6 + 6×5 = 54 Kg.m/s
Momentum after collision: p2 = (4 + 6) v2 ; v2 is the velocity of the two objects together after collision
momentum is conserved: 54 = 10 v2
v2 = 5.4 m/s
Kinetic energy before collision: K1 = (1/2) (4) 6² + (1/2) (6) 5² = 147 J
Kinetic energy after collision: K2 = (1/2) (4 + 6) 5.4² = 145.8 J
Change in kinetic energy: K2 - K1 = 145.8 - 147 = - 1.2 J
1.2 J of kinetic energy was lost.
Answer: A

Momentum before collision: p1 = 0 Kg.m/s (both objects at rest)
We assume an object moving to the right is moving in the positive direction.
Momentum after collision: p2 = 1 (-9) + 3 (v2) = - 9 + 3 v2; v2 is the velocity of object B
momentum is conserved: 0 = - 9 + 3 v2
v2 = 3 m/s and B moving to the right
Answer: B

Answer: D

Assuming positive motion when an object moving to the right. Since both objects moving along a straight line, the velocities have one component along the path of motion which a straight line and this component is either positive (to the right) or negative (to the left).
momentum before collision: p1 = m1 |v1| - m2 |v2| , |v2| the magnitude of object B.
momentum after collision: p2 = 0 (they both stop hence velocities equal to 0 after collision).
conservation of momentum: m1 |v1| - m2 |v2| = 0
Solve for |v2|
|v2| = |v1| (m1 / m2)
Answer: B